Quantum Mechanics

Table of Contents

2. A First Approach to Classical Mechanics

2.5 Poisson Brackets and Hamiltonian Mechanics

Let $f$ and $g$ be two smooth functions on $\mathbb{R}^{2n}$, where an element of $\mathbb{R}^{2n}$ is thought of as a pair $(\mathbf{x}, \mathbf{p})$, with

  • $\mathbf{x} \in \mathbb{R}^n$ representing position of a particle $\mathbf{p} \in \mathbb{R}^n$ representing the momentum of a particle

Then the Poisson bracket of $f$ and $g$, denoted $\pb{f}{g}$ is the function on $\mathbb{R}^{2n}$ given by

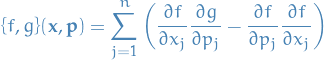
\begin{equation*} \pb{f}{g} (\mathbf{x}, \mathbf{p}) = \sum_{j=1}^{n} \bigg( \frac{\partial f}{\partial x_j} \frac{\partial g}{\partial p_j} - \frac{\partial f}{\partial p_j} \frac{\partial f}{\partial x_j} \bigg) \end{equation*}

For all smooth functions $f$, $g$ and $h$ on $\mathbb{R}^{2n}$ we have the following:

  1. $\pb{f}{g + ch} = \pb{f}{g} + c \pb{f}{h}$ for all $c \in \mathbb{R}$
  2. $\pb{g}{f} = - \pb{f}{g}$
  3. $\pb{f}{gh} = \pb{f}{g} h + g \pb{f}{h}$

  4. Jacobi identity:

    \begin{equation*} \pb{f}{\pb{g}{h}} + \pb{h}{\pb{f}{g}} + \pb{g}{\pb{h}{f}} = 0 \end{equation*}

The position and momentum functions satisfy the following Poisson bracket relations:

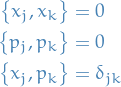
\begin{equation*} \begin{split} \pb{x_j}{x_k} &= 0 \\ \pb{p_j}{p_k} &= 0 \\ \pb{x_j}{p_k} &= \delta_{jk} \end{split} \end{equation*}

If a particle in $\mathbb{R}^n$ has the usual sort of energy function (kinetic energy plus potential energy), we have

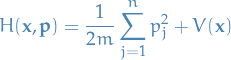
\begin{equation*} H(\mathbf{x}, \mathbf{p}) = \frac{1}{2m} \sum_{j=1}^{n} p_j^2 + V(\mathbf{x}) \end{equation*}

Wit the Hamiltonian, and as usual, having $p_j = m_j \dot{x}_j$, we can write Netwon's laws as:

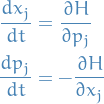
\begin{equation*} \begin{split} \frac{d x_j}{dt} &= \frac{\partial H}{\partial p_j} \\ \frac{d p_j}{dt} &= - \frac{\partial H}{\partial x_j} \end{split} \end{equation*}

These equations we refer to has Hamilton's equations.

If $\big( \mathbf{x}(t), \mathbf{p}(t) \big)$ is a solution of the Hamilton's equation, then for any function $f$ on $\mathbb{R}^{2n}$, we have

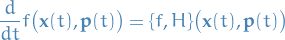
\begin{equation*} \frac{d}{dt} f \big( \mathbf{x}(t), \mathbf{p}(t) \big) = \pb{f}{H} \big( \mathbf{x}(t), \mathbf{p}(t) \big) \end{equation*}

Call a smooth function $f$ on $\mathbb{R}^{2n}$ a conserved quantity if $f \big( \mathbf{x}(t), \mathbf{p}(t) \big)$ is independent of $t$ for each solution $\big( \mathbf{x}(t), \mathbf{p}(t) \big)$ of Hamilton's equations.

Then $f$ is a conserved quantity if and only if

\begin{equation*} \pb{f}{H} = 0 \end{equation*}

In particular, the Hamiltonian $H$ is a conserved quantity.

Solving Hamilton's equatons on $\mathbb{R}^{2n}$ gives rise to a flow on $\mathbb{R}^{2n}$, that is, a family $\Phi_t$ of diffeomorphisms of $\mathbb{R}^{2n}$, where $\Phi_t(\mathbf{x}, \mathbf{p})$ is equal to the solution at time $t$ of Hamilton's equations with initial conditions $(\mathbf{x}, \mathbf{p})$.

Since it is possible (depending on the choice of potential function $v$ ) that a particle can escape to infinity in finite time, the maps $\Phi_t$ are not necessarily defined on all of $\mathbb{R}^{2n}$, but only on some subset therof.

If $\Phi_t$ is defined on all of $\mathbb{R}^{2n}$ we say it's complete.

The flow associated with Hamilton's equations, for an arbitrary Hamitonian function $H$, preserves the (2n)-dimensional volume measure

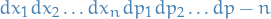
\begin{equation*} dx_1 dx_2 \dots dx_n dp_1 dp_2 \dots dp-n \end{equation*}

What this means, more precisely, is that if a measurable set $E$ is contained in the domain of $\Phi_t$ for some $t \in \mathbb{R}$, then the volume of $\Phi_t(E)$ is equal to the volume of $E$.

3. A First Approach to Quantum Mechanics

3.2 A Few Words About Operators and Their Adjoints