Electrodynamics
Table of Contents
Equations / Theorems
Guass' Law for electrical field of closed surface

for any closed surface  and
and  is the surface charge-density.
is the surface charge-density.
Poisson's Equation
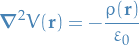
where we've used Gauss' Law and the fact that  .
.
Boundary conditions
We have the standard boundary conditions in Electrostatiscs:

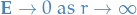
Note that since  we basically have boundary conditions for
we basically have boundary conditions for 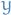 and
and  in the "normal" ODE sense.
in the "normal" ODE sense.
Properties
Uniqueness
For any two different solutions of the Poisson's equation, the solutions only differ by a constant.
Laplace's equation

which is a special case of the Poisson's Equation, where we have space absent of free charges .
Examples
The charge density within the follow region is  , and thus we have the Laplace's equation with the boundary conditions
, and thus we have the Laplace's equation with the boundary conditions  where
where  , i.e. the potential at the boundary of the inner (non-charge) region is equal to the potential of the inner boundary of the conductor (since they're obviously the same boundary).
, i.e. the potential at the boundary of the inner (non-charge) region is equal to the potential of the inner boundary of the conductor (since they're obviously the same boundary).
Method of Images
- "Imagine" a charge s.t. we get a homogenous PDE (equiv. Laplace's equation)
- Solve homogenous PDE / Laplacian using [BROKEN LINK: No match for fuzzy expression: *Laplace's%20equation%20in%20cylindrical%20coordinates], i.e. assume a solution of the form

- Then find a special solution to the non-homogenous PDE
- The linear combination of these two then form the general solution to the problem
There is one issue that one my bring up; is this solution unique? As it turns out, it is!
Maxwell's equations
Gauss's law
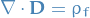
Guass's law for magnetism

Maxwell-Faraday equation

Ampère's circuital law
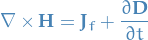
where:
 is the displacement field, which accounts for the effect of free and bound charge within materials while its sources are the free charges only
is the displacement field, which accounts for the effect of free and bound charge within materials while its sources are the free charges only is the magnetizing field
is the magnetizing field is the electric field
is the electric field is the magnetic field
is the magnetic field is the free charge density
is the free charge density